Introduction

Today, the accuracy and stability of atomic frequency standards using laser light surpass those of the cesium frequency standards defining the SI (International System of Units) second. The Space-Time Standards lab at NICT strives to further improve their performance and to apply such standards to generate a more accurate reference time. Our work has experimentally confirmed the statement "time passes faster at higher elevation" of Einstein's Theory of Relativity by direct frequency comparison between distant optical lattice clocks. In cooperation with groups in Europe and the United States, we have recently used the atomic frequency standard to search for dark matter, which is considered essential to the present understanding of the universe.

Strontium optical lattice clock at NICT

Atomic frequency standard

All atomic frequency standards rely on the characteristics of an atom during the absorption and emission of electromagnetic radiation (whether radio wave or light) at specific frequencies. Each of these transition frequencies corresponds to a change from one atomic energy level to another. The SI second is defined by a microwave transition of the cesium atom. Cesium frequency standards (cesium atomic clocks) realize this definition. The best among these standards are internationally recognized as primary frequency standards and some of them are accurate enough to not lose or gain even one second in 300 million years. This is comparable to measuring the diameter of Earth with an uncertainty of about one nanometer, which is one billionth of a meter (Fig. 1).

To improve this performance even further, we are developing an optical clock, an atomic frequency standard using laser light, which is an electromagnetic wave with a frequency higher than that of microwaves. The more frequent oscillations of light help determine the duration of one second with higher precision than possible with microwaves, in the same way that a finer scale helps measure length with higher precision. Recently developed optical clocks have been reported to have an accuracy of within one second in 16 billion years. To make this accuracy accessible within the SI system, the International Committee of Weights and Measures (CIPM) is now considering a new definition of the second based on optical transitions of atoms.

Figure 1. The accuracy of the current primary frequency standards is equivalent to measuring the diameter of Earth with an accuracy of about one nanometer, but optical frequency standards can be one or two orders of magnitude more accurate.

Single trapped ion optical clock and optical lattice clock

Today, the two schemes shown in Fig. 2 are the main schemes of optical frequency standards. One of them is the single trapped ion optical clock proposed in Europe and the United States in the 1980s. This scheme uses a single ion trapped by the Coulomb force that the ion experiences in the AC electric field generated by carefully arranged electrodes.

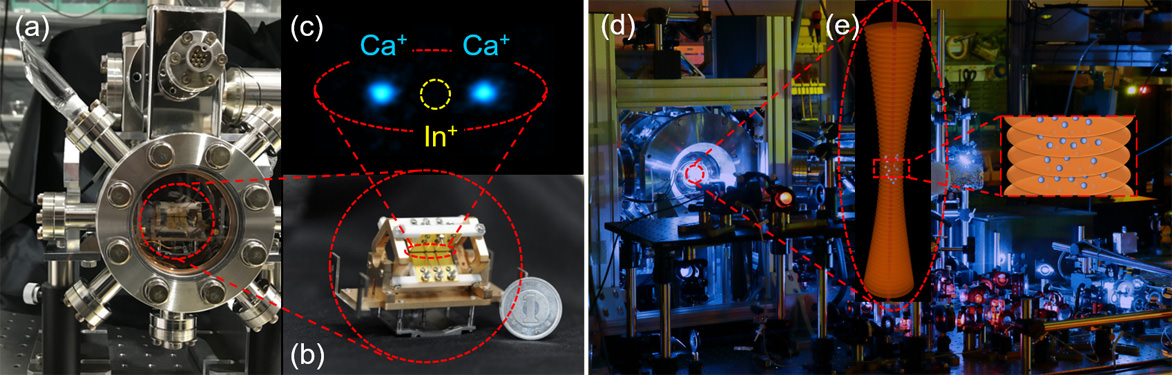
Figure 2. (a) Part of a single trapped ion optical clock, (b) photograph of the trapping electrode, (c) the single indium ion (In⁺) in the optical atomic clock is trapped between two calcium ions (Ca⁺) shining blue in the CCD camera image. (d) Part of a strontium (Sr) optical lattice clock and (e) schematic diagram of the optical lattice trapping a large number of atoms.

The other scheme is the optical lattice clock (Figs. 2 and 3) proposed by Hidetoshi Katori in 2001 when he was an assistant professor at the University of Tokyo. It uses tens of thousands of atoms trapped in the interference pattern of overlapping laser beams, a fringelike structure that has come to be described as an optical lattice. The physical phenomenon called the Stark effect causes atoms to gather at high electric field intensities when appropriate laser frequencies are selected. In today's lattice clocks, opposing laser beams create intensified or weakened regions closely spaced like a stack of pancakes. Dispersed over these regions, many atoms can be handled and probed at the same time, which provides higher signal intensity and consequently enables faster measurement than in the single trapped ion optical clock.

In our laboratory, researchers cooperate in their work on both optical lattice clocks and ion optical clocks.

Figure 3. Part of laser light sources used to cool atoms before they can be trapped in the optical lattice.

Absolute frequency measurement and direct frequency comparison of strontium optical lattice clocks

An absolute frequency is measured by referring to the frequency of the cesium microwave transition that defines the SI second. For the optical lattice clocks with strontium (Sr) atoms developed by national metrological institutes in various countries, such measurements show highly consistent results (Fig. 4).

When this is the case, the CIPM, through a proposal from the Consultative Committee for Time and Frequency (CCTF), endorses such frequency standards as secondary representations of the second with a specific recommended standard frequency. These secondary representations of the second are the best candidates for a future redefinition of the second.

Alignment of laser beams entering a vacuum chamber

Figure 4 shows the results of the most recent absolute frequency measurements of Sr optical lattice clocks. The frequency recommended for the use of these clocks as secondary representations of the second is shown by the blue line. The green line shows the new value proposed by the CCTF meeting in 2021. Our results, shown as red circles, have also contributed to the determination of these values.

The uncertainty of the absolute frequency is now limited by that of the cesium primary frequency standard used to realize the definition of the second. To evaluate the optical frequency standard more precisely, the concept of an absolute frequency can be bypassed completely by directly comparing the dimensionless frequency ratio of optical frequency standards. For this purpose, we compare the Sr standard developed at NICT with the optical frequency standards developed at other institutes using optical fibers and satellite links.
August 2011 Press Release: https://www.nict.go.jp/press/2011/08/04-1.html
May 2014 Press Release: https://www.nict.go.jp/press/2014/05/27-1.html

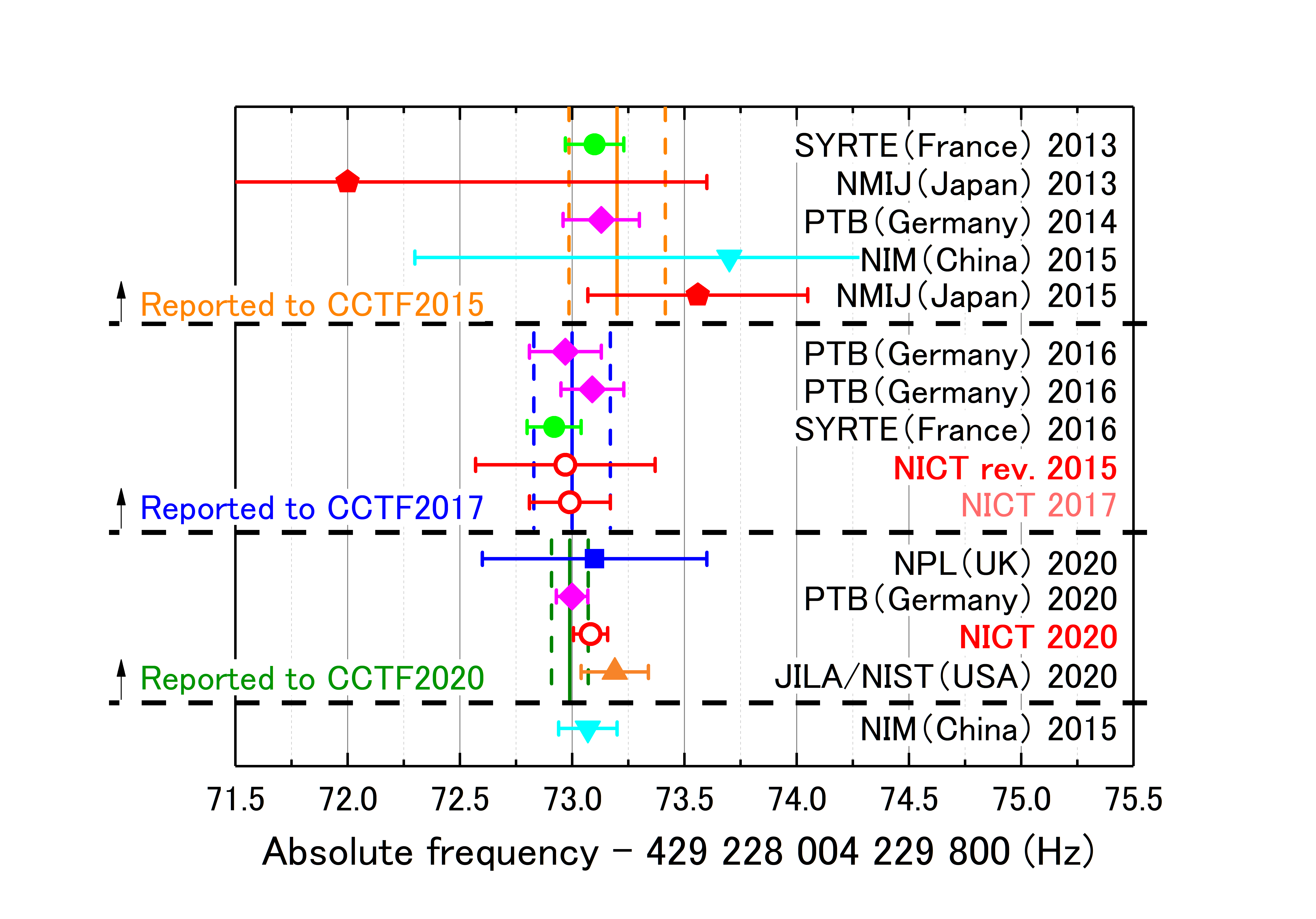
Figure 4. Absolute frequencies of Sr optical lattice clock standards at different institutes reported since 2013. The solid orange, blue, and green lines represent the recommended frequencies of the Sr optical lattice clocks as determined by CCTF meetings in 2015, 2017, and 2021. The broken lines represent their uncertainties. The uncertainty for Sr optical lattice clock is the smallest among the secondary representations of the second.

Calibration of Coordinated Universal Time with an optical lattice clock

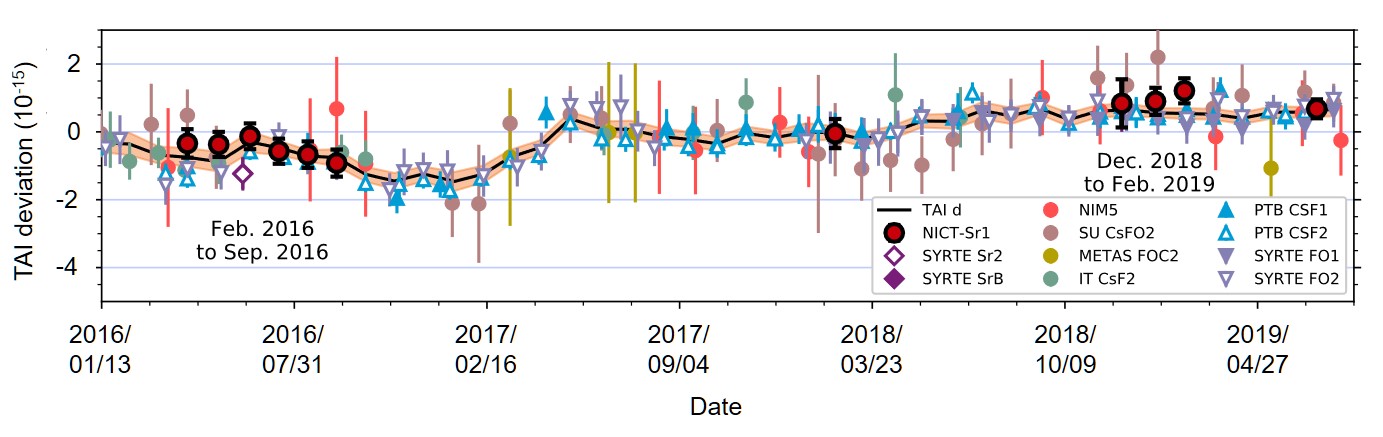
In the real world, technical developments such as global financial transactions and 5G systems make accurate time information increasingly important. The Coordinated Universal Time (UTC) provides the required international time scale. It is calculated by the International Bureau of Weights and Measures (BIPM) from the weighted frequency average of more than 400 microwave atomic clocks around the world. Primary and secondary frequency standards in many countries confirm that this time scale progresses with one tick for each precisely determined SI second. BIPM uses the results of these calibrations to correct the frequency if needed. The CCTF Working Group on Primary and Secondary Frequency Standards also selects the frequency standards considered suitable for contribution to the calibration. The Sr optical lattice clock developed at NICT was accepted as a contributing secondary frequency standard, one working as an element that is not cesium, in November 2018. Together with another Sr optical lattice clock developed at Observatoire de Paris, it became the first optical frequency standard that contributed on-time data to the steering of UTC. Except for some interruptions, it has continued its contributions since then (Fig. 5).
February 2019 Press Release: https://www.nict.go.jp/press/2019/02/07-1.html

Figure 5. To calibrate UTC, primary and secondary frequency standards measure the scale interval of the underlying International Atomic Time (TAI). This has the same frequency as UTC, which differs only by the addition of currently 37 accumulated leap seconds. The figure shows the results reported from January 2016 to July 2019. Error bars indicate the uncertainty of the measurements. The additional uncertainties for the frequencies of non-cesium transitions investigated by secondary frequency standards have been omitted here.

Generating accurate real-time signals of a local time scale

UTC as a time scale exists only in the calculations performed by BIPM once a month. It is not suitable for practical use, since no actual signal is generated. Individual countries then generate and maintain time scale signals synchronized with UTC. The signal generated by NICT usually keeps within one fifty millionth of second of UTC, and it is made widely available as Japan Standard Time with the addition of a time zone difference of +9 hours.

In 2016, our laboratory was the first in the world to demonstrate a more accurate real-time signal based on a time scale created with an optical clock. Such clocks, and optical lattice clocks in particular, are complex devices and their long-term continuous operation remains difficult. Therefore, a commercial hydrogen maser is used as a frequency source. This is another form of a microwave atomic clock, capable of stable continuous operation, even though it is not sufficiently accurate to generate a time scale by itself. The frequency of the hydrogen maser is measured and consequently corrected by operating the optical lattice clock for about three hours once a week. In this way, an actual atomic time signal TA(Sr) was generated over five months. As shown by the blue line in Fig. 6, the time difference between TA(Sr) and UTC is adjusted to 0 at the start of the comparison. The difference then gradually increases to slightly more than 8 ns (where one nanosecond is one billionth of a second) after five months.

However, even UTC is not perfect, and once a year, BIPM revisits all available data and calculates a more accurate time scale of TT(BIPM) as an implementation of the Terrestrial Time used in astronomy and other applications that require long-term frequency stability. Compared with this time scale, the deviation of TA(Sr) remains less than 2 ns over the period of five months (red line in Fig. 6). The higher accuracy of TT(BIPM) reflects that data for the full year are available at the time of calculation, whereas the more frequently updated UTC can only account for measurement up to the time of calculation. Our results show that over five months, the ticks of TA(Sr) more closely correspond to the defining one-second interval than the ticks of UTC.

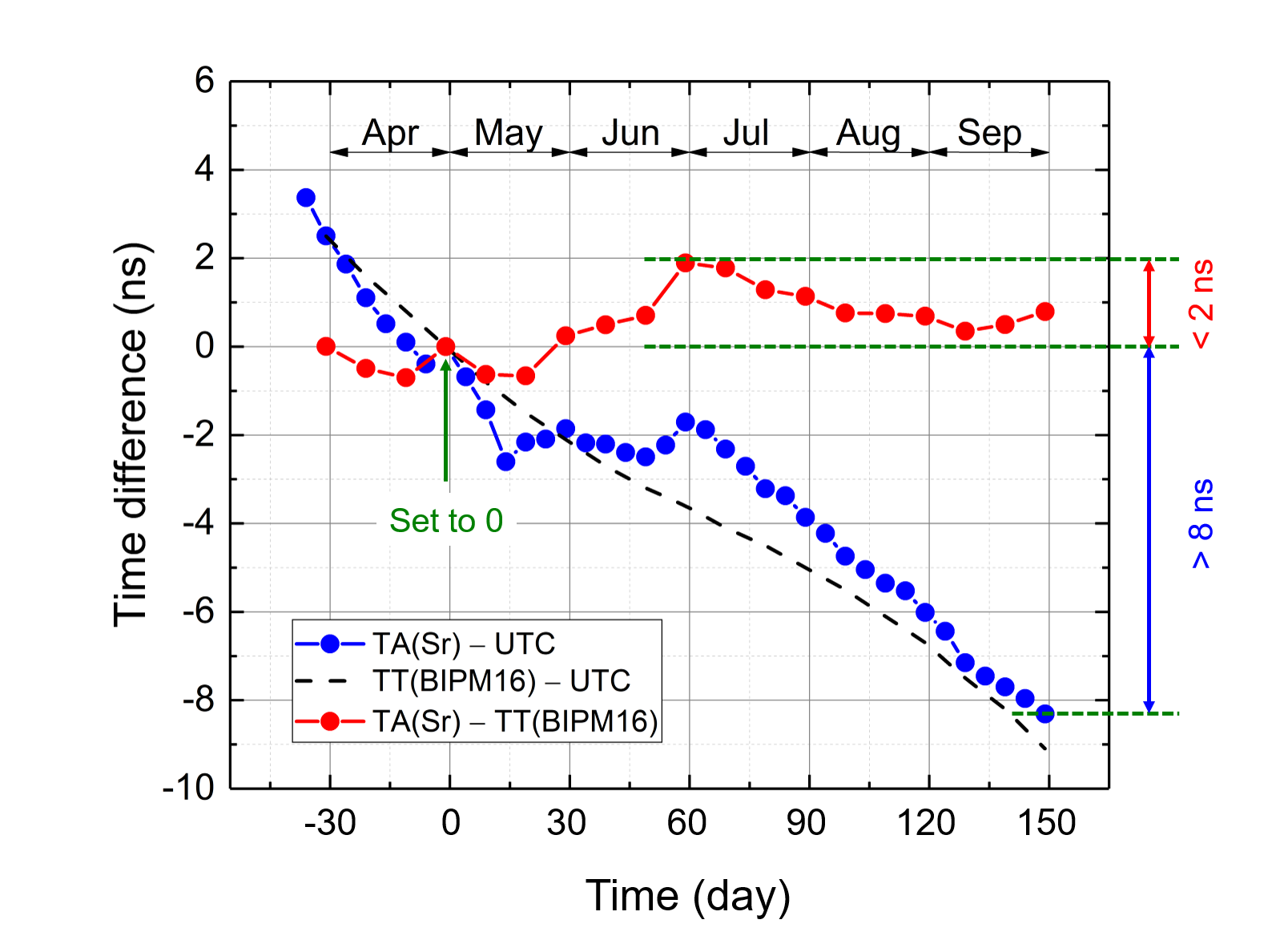
Figure 6. Accuracy of the time scale TA(Sr) generated in real-time using an optical lattice clock. The blue and red lines represent the time differences between TA(Sr) and UTC and between TA(Sr) and TT(BIPM), respectively, in nanoseconds. The time difference was adjusted to 0 at the beginning of May. The black dashed line represents the time difference between TT(BIPM) and UTC.

Fundamental science with optical lattice clocks

The theory of general relativity states that the stronger the gravitational field, the slower time passes. For us on Earth, this means that time passes faster at high altitudes. Even early in the development of optical lattice clocks, their accuracy was already sufficient to demonstrate this effect. In 2011, we used signals transmitted by optical fiber to measure the frequency difference between the optical lattice clocks at NICT headquarters in Koganei and the Katori Laboratory at the University of Tokyo, Hongo Campus. The difference directly shows that time passed faster at NICT headquarters, at an altitude 56 m higher than that at the University of Tokyo.
August 2011 Press Release: https://www.nict.go.jp/press/2011/08/04-1.html

In the future, we may be able to investigate the existence of underground cavities and resources by detecting even the most minute frequency shifts of optical lattice clocks due to differences in gravitational potential.

We have also begun to investigate the use of optical lattice clocks to explore the presence of dark matter, a mysterious substance that is believed to make up the majority of mass in the universe, even though its nature remains unidentified. In this investigation, our group participates in an international measurement project led by researchers of the Nicolaus Copernicus University in Poland [P. Wcis?o, et al., Sci. Adv. 4, eaau4869 (2018)]

Summary

Studying the interactions between an atom and a laser beam makes relativistic effects directly accessible within the space of a small laboratory. It lets us tackle grand research themes on the mysteries of the universe. This is what makes research on frequency standards attractive even beyond the quest for the ideal second.

We are already contributing to an increased accuracy of UTC by using optical frequency standards. Now, we work to improve the Japan Standard Time system, so that a more accurate time scale can be realized by using optical frequency standards. In addition, we hope to contribute to a deeper understanding of nature through the accuracy of optical frequency standards.

In the Space-Time Standards Laboratory